Consider the model shown in Fig. 1, which was used by Isaac Newton in first defining viscosity. It shows two parallel planes of fluid of area A separated by a distance dx and moving in the same direction at different velocities V1 and V2.
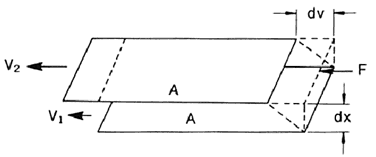
Fig. 1
Where n constant for a given liquid and is called its viscosity.
The velocity gradient,
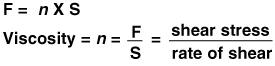
Fig. 2 shows graphically the relationships between shear Stress (F,) rate of shear (S,) and viscosity (n) for a Newtonian liquid. The viscosity remains constant as shown in sketch 2, and in absolute units, the viscosity is the inverse slope of the line in sketch 1. Water and light oils are good examples of Newtonian liquids.
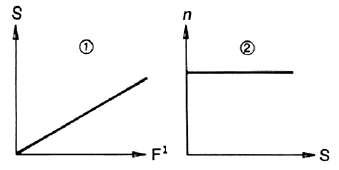
Fig. 2 Newtonian Liquid


